In pharmaceutical calculations, percentage solutions and allegations are commonly used for various purposes, including determining concentrations, preparing solutions, and compounding medications. Here’s an overview of calculations involving percentage solutions and allegations:
Percentage Solutions
Calculation of Percentage:
The percentage is calculated as (part/whole) 100.
For example, to calculate the percentage concentration of a drug in a solution:
Percentage = (amount of drug / total volume of solution ) × 100
Converting Percentage to a Ratio or Fraction:
Percentage can be converted to a ratio or fraction by dividing the percentage value by 100.
For example, 5% becomes 5/100 or 0.05.
Dilution of Percentage Solutions:
When diluting a solution to a lower concentration, use the formula:
C1V1 = C2 V2
C1 and V1 are the initial concentration and volume, C2 and V2 are the final concentration and volume.
Allegation (Alligation)
Simple Allegation:
Allegation is a method of solving problems that involves mixing two solutions of different concentrations to obtain a desired concentration.
For example, suppose you have two solutions, one with a higher concentration (H) and one with a lower concentration (L). In that case, you can use the allegation formula to find the ratio of the two solutions needed to obtain the desired concentration (D):
D – H/ L –D = H – D / D – L
Weighted Allegation:
Weighted allegation is used when the quantities of the mixed solutions are not equal.
For example, if you are mixing two solutions with different quantities to obtain a desired concentration, the weighted allegation formula is:
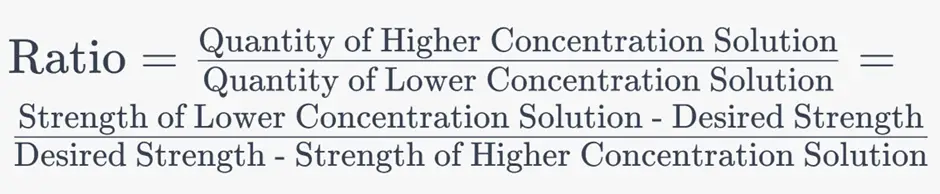
Example:
Let’s say you have a 10% solution and a 5% solution, and you want to mix them to obtain a 7% solution:
7 – 10 / 5 – 7 = 10 – 7 / 7 – 5
Solving this equation will give you the ratio in which the two solutions should be mixed to achieve the desired concentration.
These calculations are essential in pharmacy practice, especially when compounding medications or preparing solutions with specific concentrations. Always ensure that units are consistent, and double-check your calculations to prevent errors.


